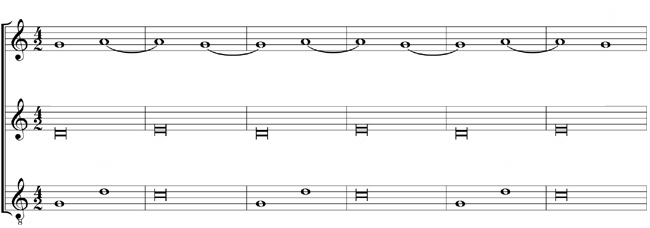
In his second letter to Cipriano de Rore, Benedetti gave the progression shown in Ex. 1a, and demonstrated, as illustrated here in Blackwood-style numeric annotations, that using Just ratios would cause the pitch to rise by a comma for each repetition of the two-measure pattern. I use the term “puzzle” for this and Benedetti’s other progression since we are clearly left to wonder what the “solution” is if these passages are not to migrate microtonally. (The sound of all migrating examples is set to loop so that listeners can hear again where the passage started in terms of pitch.)
| Ex. 1a. Benedetti’s first passage in his own version |
 |
This ascent results because the singer of the bottom voice tries to make the C at the beginning of bars 2, 4, and 6, pure as a minor third (or major sixth) against the A tied over from the previous bar in the top voice. This necessitates raising the C by one comma, which necessitates raising the G in the top voice in order to be pure to the sustained C in the bottom, and so forth. There is no question that the consistent application of the theoretical ratios to the notes in this passage would result in the comma ascent described by Benedetti and the presumptive conclusion that Just intonation is impractical. It did not go away, however, so the problem of comma migration continued to plague theorists for a long time. Christian Huygens, at the end of the 17th century, noted:
For if you ask any of our Musicians, why two or more perfect fifths cannot be us’d regularly in composition; some say ’tis … because when you pass from one perfect fifth to another, there is such a change made as immediately alters your Key, you are got into a new Key before the Ear is prepared for it, and the more perfect Chords you use of the same kind in Consecution, by so much the more you offend the Ear by these abrupt Changes.…
I say therefore, if any Persons strike those Sounds which the Musicians distinguish by these Letters, C, F, D, G, C, by these agreeable Intervals, altogether, perfect, interchangable, ascending and descending with the Voice: Now this latter sound C will be one Comma, or very small portion lower than the first sounding of C [as follows:]
 |
|
|
Therefore we are compell’d to use an occult Temperament, and to sing these imperfect Intervals, from doing which less offence arises.Fn17
The comma descent occurs, of course, because of the wide pure minor third from F to D. In the 18th-century, the theorist Robert Smith cited Huygens’ comments on comma migration, along with a revealing story printed in France in 1707. It refers to a real situation like the theoretical one described by Benedetti in his second example below:
This is also confirmed by what we are told of a monk, who found, by subtracting all the ascents of the voice in a certain chant from all its descents, that the latter exceeding the former by two commas: so that if the ascents and the descents were constantly made by perfect intervals, and the chant were repeated but four or five times, the final sound, which in that chant should be about the same as the initial, would fall about a whole tone below it. But finding that the voices in his quire did not vary from the pitch assumed, he concluded that the musical ratios, whereby he measured those successive ascents and descents were erroneous.Fn18
The monk’s example appears to be a strophic piece which, technically, should have migrated downward by two commas each time through. Thus, “four or five times” through the music, starting each stanza on the ending pitch of the previous one, would cause the piece to drop a total of eight or ten commas respectively (the whole tone being made up of nine commas). Since the piece didn’t descend as predicted, the monk decided that the harmonic ratios must have been erroneous, but we know they were not: it was some basic “homing instinct” on the part of the singers to maintain their original pitch level. Frequently in the Renaissance, furthermore, choirs sang in alternatim with organ, making a stable pitch not just desirable but necessary.
Indeed, Benedetti notes that the migration problem does not occur on keyboard instruments, and recommends a tuning system with tempered fifths ascending to G# and descending to Eb. Claude Palisca says,
Benedetti did not say that his tuning was an equal temperament, but since his demonstrations show that all semitones and whole tones should be equalized, this would have been a logical goal. Indeed, his tuning method is not unlike that proposed by Giovanni Lanfranco in 1533, which J. Murray Barbour has interpreted as equal temperament.Fn19
The method of tempering fifths up to G# and down to Eb is a classic meantone procedure, however, and in any case, Mark Lindley disputes Barbour’s characterization of Lanfranco’s tuning as equal temperament, seeing, rather, a mild meantone such as one-fifth comma.Fn20 (Meantone temperaments distribute fractions of the syntonic comma among the various fifths in the system. They also feature major and minor semitones, although their whole tones are standard within each system.) Indeed, beginning with the instructions given by Arnolt Schlick in 1511,Fn21 theorists had begun to include various forms of meantone in their—mostly vernacular—discussions of tuning. It is always clear, however, that such systems are intended for the use of instruments rather than singers. Schlick’s system seems to be fifth- or sixth-comma meantone. Aaron’s system from his Toscanello (1523)Fn22 is thought by many to have been quarter-comma meantone (the most famous form of meantone with resulting pure major thirds). Zarlino recommended two-sevenths-comma meantone in 1558 and changed to quarter-comma in 1571. Francisco de Salinas in 1577Fn23 discussed quarter-comma and also recommended third-comma meantone (which has the added advantage that it works as a nineteen-note equal temperament). In fact, quarter-comma works as an equal temperament approximation as well, with 31 notes to the octave, and this is essentially the system proposed by Vicentino for his arcicembalo in 1555.Fn24
Meantone is certainly one possible “solution” to these tuning puzzles, although all of the fifths are quite narrow in anything approaching the quarter-comma variety, and that’s why people continued to look for “just” solutions that provide at least some places of repose. Here is Benedetti’s passage in a succession of meantone temperaments.Fn25
|
Ex. 1b. Benedetti’s first passage in various regular temperaments |
 |
|
1/3 |
|
2/7 |
|
1/4 |
|
1/5 |
| 1/6 |
|
ET
|
Any choice of meantone temperament is a trade-off between musical quality and convenience. The most extreme form given here—1/3 comma—has pure minor thirds, which means that it sounds especially good in modes and pieces that emphasize minor triads. It can also be used in a 19-note octave to create an extended meantone system. Its drawbacks, however, include a a major third that is narrower than pure, and a fifth that is extremely narrow. Listeners can hear in the 1/3 comma version how the odd-numbered measures sound sour since they contain only open fifths, whereas the even-numbered measures are somewhat better. The 2/7 comma version sounds similar but slightly better with the improved fifths and major thirds, and the version in 1/4 comma meantone sounds better still in the open fifths (though not actually pleasant) and very euphonious in the triads. In 1/5 comma meantone, the fifths and major thirds are about the same distance from pure (though the third is wide and the fifth narrow), which means that they beat at a similar rate and create a kind of “vibrato” effect that some listeners find attractive. The 1/6 comma version is slightly better in the fifths but slightly worse in the major thirds, though they are still quite acceptable—a good compromise, perhaps. (The virtues of 1/6 comma meantone become evident with more complex harmonies.)Fn26 Finally, the equal tempered version exhibits excellent odd-numbered measures because its fifths are almost pure, but its major thirds are excruciatingly wide, causing the even-numbered measures to sound very sour and “jangly.”
While the use of any temperament would solve the migration problem, and while singers can learn to adjust to meantone when they are obliged to sing with a keyboard, it seems very unlikely that these are systems that Renaissance singers, left to their own devices and singing unaccompanied, would conceive for themselves. Furthermore, I have yet to discover a single Renaissance pedagogue recommending such temperaments for singers. Even Benedetti’s recommendations, given after pointing out the difficulty of maintaining Just intonation, are for tempering “in organis & clavicymbalis.”Fn27 And it is difficult to imagine that singers would look at his temperament recipe and say, “Oh dear, I won’t be able to sing that Ab in tune because I’ve only got a G#;” or “It’s too bad we can’t sing that fifth pure.” In my experience, that’s not the way singers think.
One thing about the use of Just tuning in Ex. 1 is that Benedetti assumes that the pitch of any note would not change throughout its duration and, while this seems like a reasonable supposition, we don’t know for sure, and Benedetti’s score (see Plate 1) with tied notes like modern notation, could be interpreted to invite that possibility.
|
Plate 1. Benedetti’s two tuning puzzles, from Diversum, pp. 279, 280 |
 |
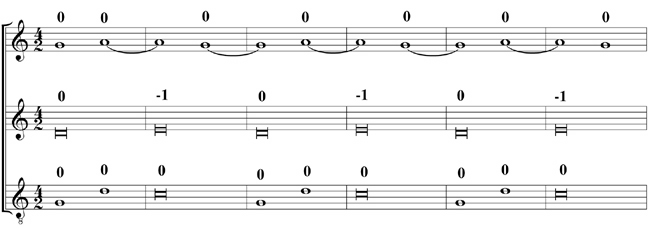
Such changing pitches have been the basis for the Just tuning solutions of Easley Blackwood.Fn28 With apologies to Prof. Blackwood, I think he would try to resolve Benedetti’s tuning puzzle as shown in Ex. 1c:
| Ex. 1c. Benedetti’s first passage in a Blackwood version |
 |
|
|
As may be seen, Blackwood’s approach is to allow the pitch of a note to change, so that it starts at one pitch but adjusts by a comma part way through. Thus, the A in the top part starts at 0 in order to be pure to the D0 below it, but it changes to –1 in order to make a pure minor third (or rather, major sixth) to the C0 at the beginning of bar 2. Meanwhile, the E is at –1 to make a pure major third above the C. All of this allows the G in the top part in bar 2 to stay at 0 against the C0 and thus prevents the microtonal modulation of Benedetti’s version.
I think the mere fact that Benedetti pointed out complications in the use of Just intonation and gave examples actually supports the case for the its use in Renaissance polyphony generally. Since he was aware of where the system is vulnerable, he must have been very familiar with it. I also recognize the theoretical correctness of Benedetti’s version, but in general I agree with Blackwood that while such microtonal modulation may be unavoidable in some cases, it is undesirable in most situations. It is especially hard to imagine real musicians being tempted to adopt a solution that results in progressive departure from the starting pitch.
While the monk’s singers in the story given above help to confirm that microtonal modulation is undesirable (and additionally confirm that choirs were still thinking about Just intonation in the eighteenth century), I actually find Easley Blackwood’s solutions using mid-note adjustments to be extremely awkward for performers and strange for listeners. Because of his comma shifts on suspended notes, it is frequently some harmonically and rhythmically prominent chord that becomes the conspicuous focus of the adjustment. This raises the question of whether there is a “quasi-just” solution that would maintain the overall pitch level but sacrifice the perfection of certain less prominent harmonic intervals in order to accomplish that. There are, in fact, two such possibilities in this passage. One is to sacrifice the purity of the syncopated D-A fifth in the second half of the first measure, as in Ex 1d. This allows the impure D0–A-1 fifth to occur in a rhythmically weak position, and then follows that sonority with pure first inversion and root position triads. It also allows the syncopated canon between the top two voices to use the same size of whole tone (the minor tone), so this solution has some melodic logic as well, even though the offending D0–A-1 fifth, albeit brief and unaccented, is extremely unpleasant.
| Ex. 1d. Benedetti’s first passage in Duffin version 1 |
 |
|
|
In fact, I chose the timbre for these examples because it actually seems to exaggerate the effect of the tuning. Just to show that voices in a live acoustic with vibrato would sound significantly better, I have included an alternative rendering of Ex. 1d using a voice-like timbre with vibrato and reverb.
|
|
This kind of tuning subtlety is not at all worthwhile when an extensive amount of vibrato is used, but a pitch variance of 22 cents is negligible for vibrato anyway. Moreover, using vibrato to cover up imperfections in tuning is something that happens all the time in performances today, even where the goal is Equal Temperament.
An alternative and perhaps more appealing quasi-Just solution is to sacrifice the purity of the suspended note in the second measure (see Ex. 1e). Since that note resolves downward to form a triad, it is treated like a suspended dissonance, so harmonic impurity may seem less of an issue even though the variance from the pure interval is also one comma. And in spite of the fact that the impurity occurs on a strong beat, it is not as difficult for the performer nor so jarring for the listener as Blackwood’s comma shift.
| Ex. 1e. Benedetti’s first passage in Duffin version 2 |
 |
Benedetti’s second puzzle (see Ex. 2a) shows downward migration of the pitch level, comma by comma, in the repetition of a different two-measure pattern. In Benedetti’s version, the E in the bottom part must be at –1 to be pure to the G0 in the middle part, and the C# in the top part is at –2 to be a pure major sixth above that E. The rest follows from overlapping intervals.
| Ex. 2a. Benedetti’s second passage in his own version |
 |
This passage appears to have a more obvious alternative solution than Benedetti’s first example (see Ex. 2b). Since the E in the bottom voice is dissonant with the top voice when it enters, that dissonance overshadows the need for purity in the G–E minor third. Thus, the impurity of that minor third occurring with a dissonance is inconsequential, and the rest of the notes can stay stable in pitch.
| Ex. 2b. Benedetti’s second passage in Duffin version |
 |
It also happens that G0 to C#-1 is a much better tritone than G0 to C#-2—in fact, it is the theoretically correct, pure tritone, with a ratio of 45:32 as given by several theorists—so not much, if anything, is lost on the harmonic purity side anyway. Using dissonant and unaccented sonorities to hide momentary imperfections in the tuning thus appears as a potentially fruitful approach to making Just intonation work in practice.
Footnotes- Back to main body
17. Christian Huygens, Cosmotheoros (1698), pp. 88-90. As his preferred tuning method, Huygens, in fact, recommended 31-note Equal Temperament, a system which is almost identical to extended quarter-comma meantone. Quarter-comma and other meantone temperaments are discussed below.
18. Robert Smith, Harmonics, or the Philosophy of Musical Sounds, second edition (1759), pp. 229, citing “Méthode genérale pour former les Systèmes temperés de musique,”Memoires de l’Académie des Sciences (1707), p. 263.
19. Palisca, Humanism, p. 264.
20. Mark Lindley, “Early 16th-Century Keyboard Temperaments,” Musica Disciplina 28 (1974), 149-50.
21. Arnolt Schlick, Spiegel der Orgelmacher und Organisten(Speyer, 1511), ch. 8.
22. Pietro Aaron, Toscanello (Venice, 1523), Book II, ch. 41.
23. Franciso de Salinas, De Musica Libri Septem (Salamanca, 1577), book 3.
24. See his L’Antica Musica Ridotta alla Moderna Prattica(Rome, 1555), Book V; translated by Rika Maniates asAncient Music Adapted to Modern Practice, ed. Claude V. Palisca (New Haven & London, 1996), Book V, 315–443. This is a long and complicated discussion, but on pp. 432–433, for example, he describes his whole tone as made up of five “dieses” or commas, of which three belong to the major semitone and two to the minor semitone. Thus, an octave consists of seven major semitones (7 x 3 = 21) plus five minor semitones (5 x 2 = 10), or of five whole tones (5 x 5 = 25) plus two major semitones (2 x 3 = 6), both of which methods give a total of thirty-one equal parts.
25. It is not sufficiently recognized, I believe, that each of these meantone temperaments possesses one or more Just intervals. Pythagorean tuning is known to contain pure 5ths and 4ths, of course, but each of the regular meantone temperaments given in Ex. 1b has some purity as well.
|
Regular Meantone version
|
Pure interval
|
Ratio
|
|
1/3 Comma
|
minor 3rd
|
6:5
|
|
Major 6th
|
5:3
|
|
|
2/7 Comma
|
minor semitone
|
25:24
|
|
1/4 Comma
|
Major 3rd
|
5:4
|
|
minor 6th
|
8:5
|
|
|
1/5 Comma
|
Major semitone
|
16:15
|
|
Major 7th
|
15:8
|
|
|
1/6 Comma
|
tritone
|
45:32
|
|
diminished 5th
|
64:45
|
The fact that each of these sytems found adherents in the Renaissance may have been due, in part, to the presence of these pure intervals and the consequent enhancement to certain modes and progressions.
26.See my forthcoming article and practice resource, “Baroque Ensemble Tuning in Extended 1/6 Syntonic Comma Meantone,” Digital Case (2006). Back to Fn26 main text
27. Benedetti, p. 281.
28. See his Structure, especially ch. 7.
